Home Experiments
Leonardo Da Vinci's studies on Bubble Caustics (ca. 1508)
Already Leonardo Da Vinci observed and described the cross-shaped pattern of light below a surface bubble. Although his explanation was wrong (hypothesising 4 attached smaller bubbles to be responsible for the cross), it is the oldest description of the phenomenon I have encountered so far.
see the Chapter on "Images Passing through Perception, Science and Religion" by C. Maltese in the book "Representation: Relationship Between Language And Image" (World Scientific Publishing Co. Pte. Ltd., Singapore, 1994).
Leonardo's original manuscript pages can be found in the digital e-Leo archive of The Biblioteca Leonardiana of Vinci (a sketch is shown in the Paris Manuscript F page f28v):
- https://www.leonardodigitale.com/en/browse/france-manuscript-f/0028-v/
- https://www.leonardodigitale.com/en/browse/codex-atlanticus-hoepli-ed/0075v_a-b-c/
A detailed study reveals the pattern to be a highly-complex composite light pattern consisting of several caustics (cusps, folds, astroid) and borders by aperture effects.
Gravitational lens imaging phenomenology with a simple toy model
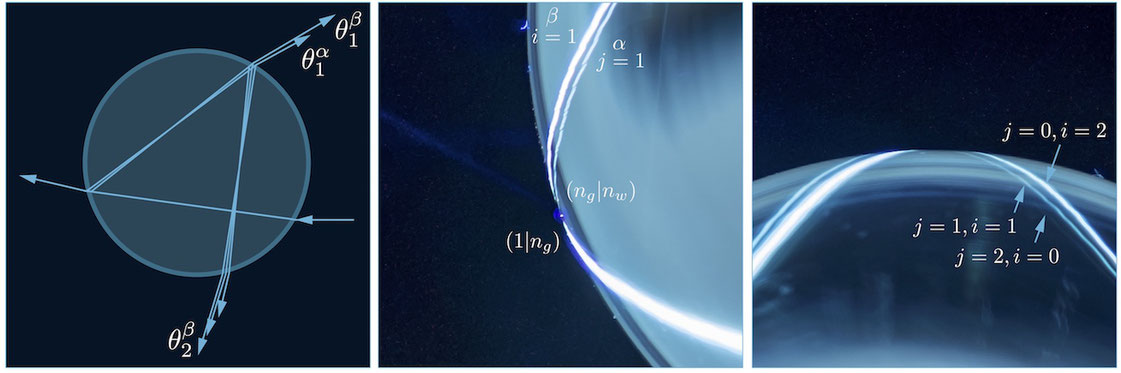
Using drawing board pins (tacks) positioned at a water-air interface and lifted by a bit produce menisci which represent axicon lenses (cf. single "bubble optics", below and under publications). Arranging one, two or more allows the reproduction of the gravitational microlensing phenomenology of n-body systems. Below are shown the results for single mass microlensing: an Einstein ring, and Einstein cross (single mass with external shear, here achieved by looking under an angle through the water meniscus lens towards the LED point light source mounted at the ceiling).
I have also recreated binary mass and triplet mass systems images.

Imaging with bubble axicons

Surface bubbles act as axicons (details in my manuscript "Bubble optics"). They have a positive refractive power combined with negative spherical aberrations, causing an axial line caustic instead of a focal point (as for a perfect regular lens). They hence represent lenses of infinite focal depth of field (DOF).
The photograph shows images of a structured light source (an LED matrix masked to show an "F" letter) placed ca. 1 m above a cereal bowl containing nothing but water. In theory, sharp images are expected to form at any depth greater than 3.3 cm from intermediate sized bubbles. At depths >5 cm virtually all bubbles form sharp images. The magnification only depends on the distance of the object, and all images are real and inverted and of equal size irrespective of the size of the bubble.
related: "Optics & Photonics News": Image of the week 19-09-16 / OSA-OPN tweet / OSA facebook post / instagram post.
Bubble caustic vs. the shadow sausage effect caustic
The shadow sausage effect (J.A. Lock at al. "Analysis of the Shadow-Sausage Effect Caustic", Appl. Optics 42(3), 418-428 (2003), available for download on Lock's homepage) is closely related to the bubble caustic. However, in detail the inversion structure of the rays forming the caustic is different from the inclined rod shadow sausage effect caustic, while it is in line with the behaviour of the vertical rod shadow sausage effect caustic. Details will be given in my manuscript "Bubble optics".


Bubble caustics
Individual air bubbles floating at a water-air interface act as axicon lenses. They have an infinite focal range and form an axial line caustic. This line caustic is structurally unstable by the theory of catastrophe optics, and unfolds into a four-cusped astroid (star-/rhombus- or needle-like) caustic (as described in appendix 2 of M.V. Berry & C. Upstill, "Catastrophe optics: morphologies of caustics and their diffraction patterns", Prog. Opt. 18, 257-346 (1980). Available for download on Berry's homepage). On a sunny day in a pool such caustics may be observed easily, see the photographs below.
In my manuscript "Bubble optics" I describe the optical properties of these special bubble lenses and their phenomenology.
Laser bathymetry of acrylic prints
"Laser bathymetry" is a term coined by the Russian physicist Lev S. Dolin for a measurement technique of shallow waters. The article "Laser bathymetry based on the halo effect", Appl. Opt. 58(6), 1555-1561 (2019), described the intensity pattern obtained by illuminating a shallow basin of water. What appears to the observer is a halo-like effect, caused by scattering of the incident beam from the bottom of the tank and the partial total internal reflection of part of the backscattered light by the water surface. This phenomenon is related to Snell's window, see for instance "Snell’s window in wavy water", Appl. Opt. 54(4), B8-B11 (2015), David K. Lynch.
Illuminating a standard commercial acrylic print with a laser pointer, the same effect can be observed. This phenomenon has indeed been discussed in an earlier publication, "Halo and subsurface scattering in the transparent coating on top of a diffusing material", Appl. Opt. 35(7), 1192-1203 (2018), L. Simonot et al.
A small Rainbow box

This rainbow box was built along the lines described in the article: "The rainbow in the box", American Journal of Physics (Am. J. Phys.), Vol. 86(4), 316-318, M. Selmke and S. Selmke, 2018.
That is, acrylic spheres of diameter 6.4mm (from Modulor) have been glued onto black carbon rods (here: diameter 0.75mm, from Conrad), arranged in a cubic volume, mounted on a hard-foam board.
Defocused image series under parallel white light illumination:

Fake vs. real caustics
The virtual halo box and wine glass caustic studies (see below) allow to show the difference between real and fake caustics. The term fake caustics has been introduced by M.V. Berry and S. Klein in the article "Diffraction near fake caustics", Eur. J. Phys. 18, 303--306 (1997), and describes caustic-equivalent patterns due to incoherent light averaging in refraction scenarios that involve rotations (or rotational sampling) of prisms and connected minimum deviation phenomena. In contrast, real caustics occur for curved interfaces and show diverging intensities in the framework of geometrical optics. See also my publication: "Artificial Halos", Am. J. Phys. 83(9), 751-760 (2015).
The difference between these two refraction phenomena can be seen in the dusty-air renderings below: The 100 hexagonal glass crystals sampled from the column orientation class (singly-oriented columns, aspect ratio c/a=2) refract the incoming parallel light into several parallel (re-directed) beams. All refractions together produce a clusterings of light rays by minimum deviation effects. Here: the lower and the upper tangent arcs represent fake caustics identifiable through the clustering of projection spots above and below the direct transmission direction. The wine glass, in contrast, shows a focusing of light. The volumetric caustic is intersected by the horizontal plane and shows a characteristic hour-glass-shape for an about half-filled typical white wine glass.
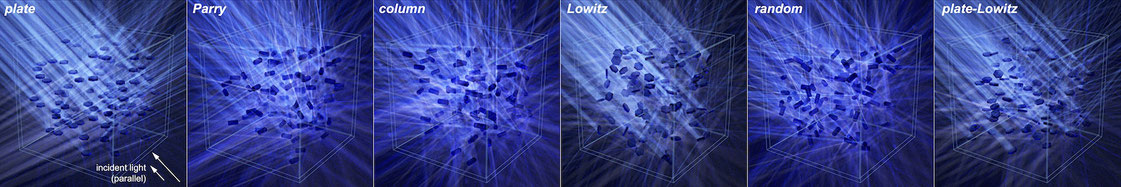
More room-corner projections of virtual halo boxes (i.e. boxes containing 100 glass (n=1.52) crystals sampled from a certain halo orientation class), as well as close-ups of the halo boxes under a slightly different viewing angle are shown below. The different scattering directions and characteristics are well visible. I.e., for plate- and Parry-oriented crystals there is a bundle of parallel rays reflected from the bottom-facing horizontal faces, resulting in a mosaic projection of a subsun (cropped from the image).
The following view gives even wider angle shots of the same scene, also showing e.g. the subsun spots:
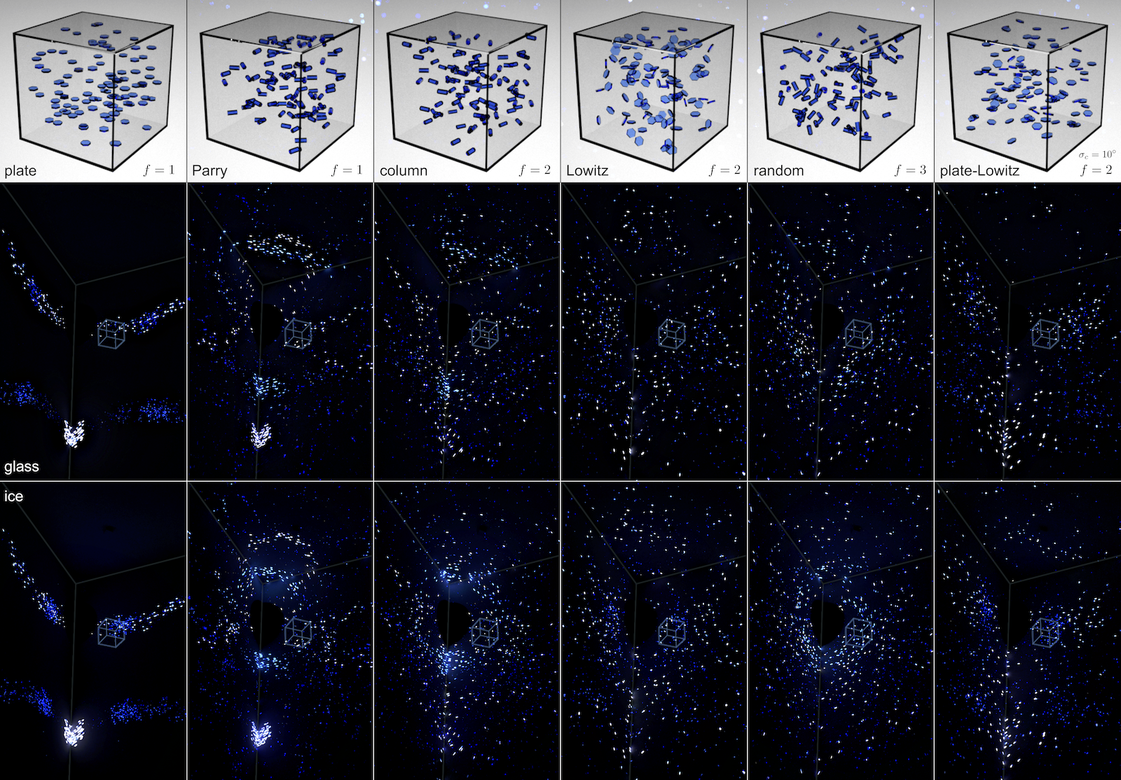
Virtual Halo boxes
Similar to the Wine glass caustic study below, the following raytracing study was inspired by Joshua S. Harvey et al.'s recent article "Bow-shaped caustics from conical prisms: a 13th-century account of rainbow formation from Robert Grosseteste’s De iride", Appl. Opt. 56(19), p.G197-204, 2017. Similar to the wine glass study, blender was used in combination with LuxRender to get accurate refractions.
In particular, the virtual Parry boxes' results nicely match the experimental patterns obtained using a physical realization (see left). The sparse spot-patterns for those orientation classes with more than 1 degree of freedom (f) yield less easily identifiable halo patterns. Only the random orientation class with its associated circular (here: 39°) halo is readily and unambiguously recognizable.
The results have been described in the 2018 Appl. Opt. article "Halo in the box - a macroscopic crystal arrangement to project mosaic halos".
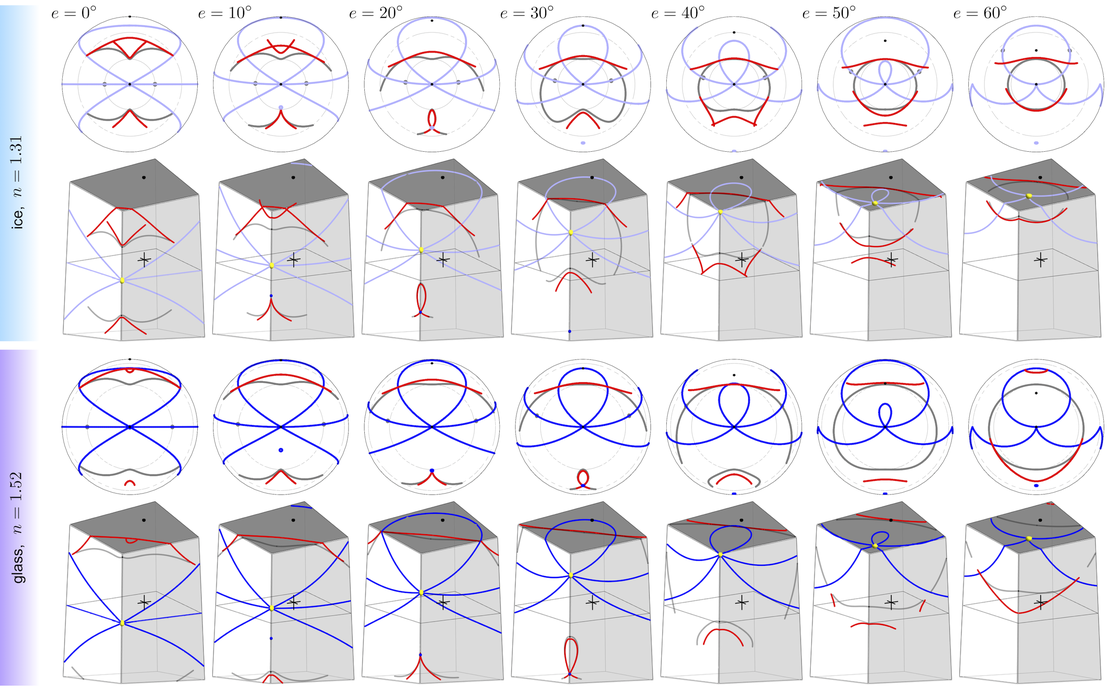
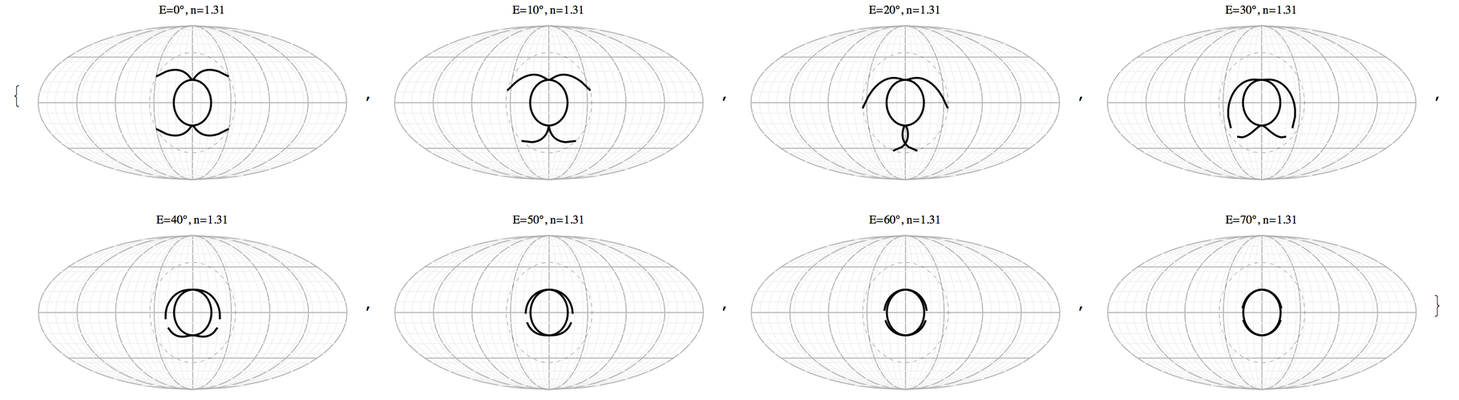
The following raytracing results show the projection patterns obtained when the shown cubic volume arrangements of hexagonal crystals (corresponding to a sampled set of 100 orientations from a given halo orientation class) are illuminated by white light. Absorption (yielding blue) allows the identification of spots made up of rays which traversed the crystals, they appear blueish. White spots correspond to outer reflections from the crystals. The two rows of projections show results for glass crystals (i.e. mimicking the experiment, n=1.52) and ice crystals (n=1.31).
The following plots show projections of the same virtual halo-boxes onto spherical screens, where the direct transmission light has also been blocked as in the above corner projections. Note however the changed order of random and Lowitz-orientations in the plots below relative to the sequence above. The views below are (nearly) orthographical onto the projection sphere and are sun-centered (i.e. the camera views the screen at an angle of 35° to the horizontal with the halo box at the view's center). Superposed are theoretical plots of the halos, i.e. the deflection functions (Parry, heliac, parhelic circle) or the corresponding fake caustics (tangent arcs, 22° circular halo, Lowitz halos).
Difference between virtual ice and glass crystals show only in the spots' positions for refraction halos, whereas the intensities are also affected for reflection halos. The following two renderings for Parry-oriented crystals using "dusty air" visualize this effect: notice how the spotted lower sunvex and the upper suncave Parry arcs (and the corresponding ray bundles, cf. red lines in plots above) move away from the forward direction as the index of refraction changes from ice (1.31) to glass (1.52).
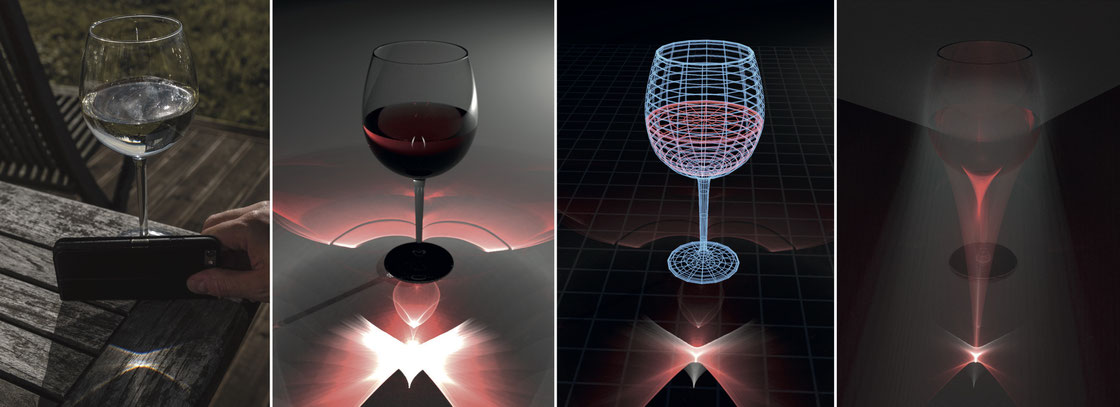
Wine glass caustic
An hour-glass-shaped caustic can be observed when a typical wine glass is illuminated under a shallow angle of about or less than 30°. The following raytracing study was inspired by Joshua S. Harvey et al.'s recent article "Bow-shaped caustics from conical prisms: a 13th-century account of rainbow formation from Robert Grosseteste’s De iride", Appl. Optics 56(19), p.G197-204, 2017. More on these investigations can be found in my article "Wine glass caustic and halo analogies", Appl. Optics 57(19), pp. 5259-5267 (2018).

Using the free 3D CAD program Blender (Blender v2.79b) in combination with the free physics-based render engine LuxRender (LuxRender 1.6.0 Build 16132, LuxCore 1.4+, LuxBlend 2.5), a ray-tracing of the scene gives remarkably similar results to the actual real-world scene for the light source inclination of 24° (corresponding to the solar elevation of the image taken in Trondheim above).
Several volumetric caustics have been made visible by adding volumetric scattering in air. Only those rays show scattering which have first interacted with any part of the geometry (i.e. liquid, glass).
Several online tutorials exist on how to model a wine-glass, e.g. here. The trick to get accurate refractions is to use LuxRender's volume precedence system. The liquid volume is not only a copy of the inner glass geometry closed off by the water-air surface (with a meniscus), but it is slighty up-scaled by 1% after modeling to have it overlap with the glass wall volume. Assigning a glass2 material linked to a glass volume with higher priority than the water volume will ensure that the part of the water that overlaps is not considered while at the same time the volumes "touch" perfectly and refractions are taken into account properly according to the two media's relative index of refraction.
Essential parts of the wine glass construction process are otherwise: a polygon segment tracing out the wine-glass exterior, use of the spin tool, removal of duplicates (vertices) to join the mesh, application of the solidify modifier to the bowl-part of the generated geometry & application of a subdivision modifier (level: 3).
The liquid volume was set to absorb and cause a reddening of white light transmitted through it. The degree of the reddening is linked to the path length within the liquid of the light rays which traverse the volume. This allows to readily identify the geometrical origin of the rays for different parts of the projected and the volumetric caustic.
The blender model / scene is available upon request.
The caustic's shape is mostly insensitive towards different wine glass geometries. However, certain details change of course. Most notably is the effect on the pattern boundary away from the stem of the glass. One may notice that rays emanate not from the entire bottom of the bowl anymore as the downwards-refracted rays passing the liquid-air interface do not reach down to the bowl's bottom. This brings the refraction scenario even closer to the analytical model described in my Applied Optics article.
Different filling levels:
Different light source elevations / inclinations towards the horizontal:
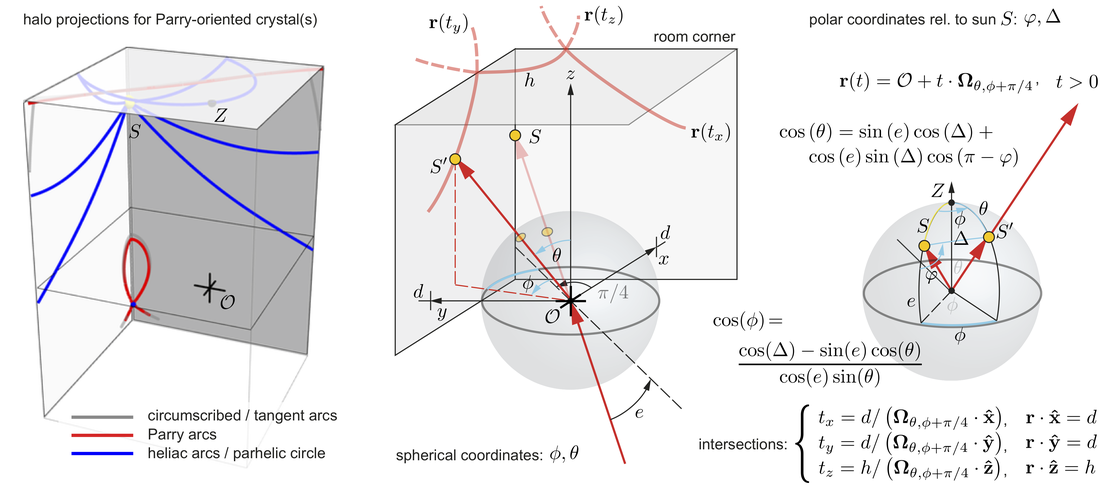
Room corner projections
Experiments using halo devices typically lack an available large spherical projection screen. An alternative yielding reasonable results can be a room corner, i.e. 3 joined perpendicular projections screens (plane z=h=const., x=d=const. and y=d=const.). The following sketch outlines the transformations needed to generate corresponding projection predictions starting from the usual polar coordinates (Δ, φ) relative to the sun S (right-most panel). The calculations involve an intermediate determination of spherical coordinates (θ, ϕ) via spherical trigonometry (law of cosine), as well as simple vector algebra to determine the ray's plane intersection. For one-dimensional halos such as the Parry arcs, the parhelic circle or the heliac arc, a single crystal orientation coordinate will then parametrize the projections in the room corner on the walls.
The following plots illustrate the differences in (sun-centered) orthographic views of spherical projections and perspective views of corresponding room corner projections for several incident light elevations e. Experimentally, the latter room-corner projections are more easy to realize. However, as the plots show, distortions cause the halo displays to deviate from the text-book renderings. The upper two rows of plots show different elevations for ice crystals (or NaF, or any material with n=1.31), while the latter two show the halo displays for glass crystals with refractive index n=1.52. The room-corner projections used d=0.5 and h=0.6, the deflections functions (Δ, φ) were taken from R.A.R. Tricker's and W.J. Humphreys' books.
Halo box - mosaic halo projections using a scattering volume
Using small hexagonal glass crystals (4 x 4 x 8 mm) positioned throughout a small cubic volume (8 x 8 x 9 cm), the following experiment represents an artificial cirrus cloud bearing halo-producing ice crystals. They have been aligned in Parry-orientations, such that they sample in a mosaic fashion the corresponding halos: heliac arcs crossing through the helic point, the parhelic circle as well as the upper suncave Parry arc (marked with an asteriks * in the sun-centered theoretical plots below) and the lower sunvex Parry arc. The latter two involve refraction and appear as colored spots on the projection screen (a corner of a room). The plots show reflection halos as blue curves, and refraction halos as red curves. The gray dashed circles represent the angles of minimum (→22° circular halo) and maximum deviation.
Flask Experiments - Laser Beam investigations
Inspired by what I have learned from writing the article "Revisiting the round bottom flask rainbow experiment" (see publications, accepted at American Journal of Physics (Am. J. Phys.), M. Selmke and S. Selmke, 2017), I have imaged the responsible ray paths directly using a 403nm UV laser diode (20mW). The round bottom (Florence) flask was water filled and imaged from above, while the laser beam was traveling through the flask equatorial plane.
Math toys for artificial halo experiments
Math toys or educational math props should be ideal for inexpensive DIY halo experiments or demonstrations in physics classes.
A google-search for "View-Thru geometric solids" / "clear geometrical solids" / "3d shapes Volume Set (fillable)" / "geometric volume / shape set" (in German: search for "Mini Füll-Körper") gives a good variety of liquid-fillable shape sets running at about 20$ / 20€ which typically contain a hexagonal prism (for parhelia & other halo phenomena), a cylinder (for analog circumzenithal / circumhorizintal halo experiments), a equilateral triangular prism (for parhelia experiments) and a cone (for analog Parry halo experiments) amongst others.
cf.: Geometriesatz (Wiemann Lehrmittel), Transparent Relational Geometric Solids (Nasco), ETA hand2mind Blue Power Solids with Lids for Volume Measurement, Learning Resources Relational Geometric Solids, Learning Advantage 21335 8 cm Geometric Volume Set (Pack of 14), ...
See my publications and experiments for details on the experiments.
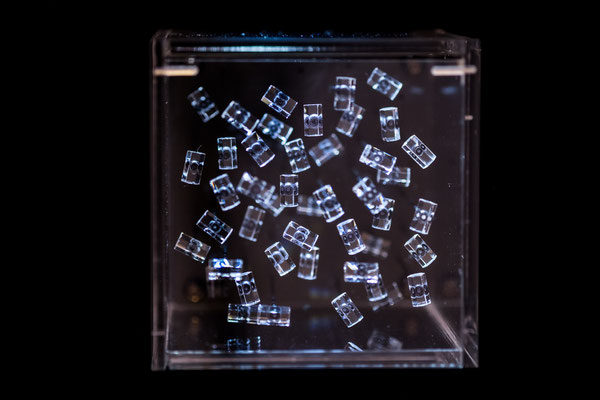
A "rainbow" in a box
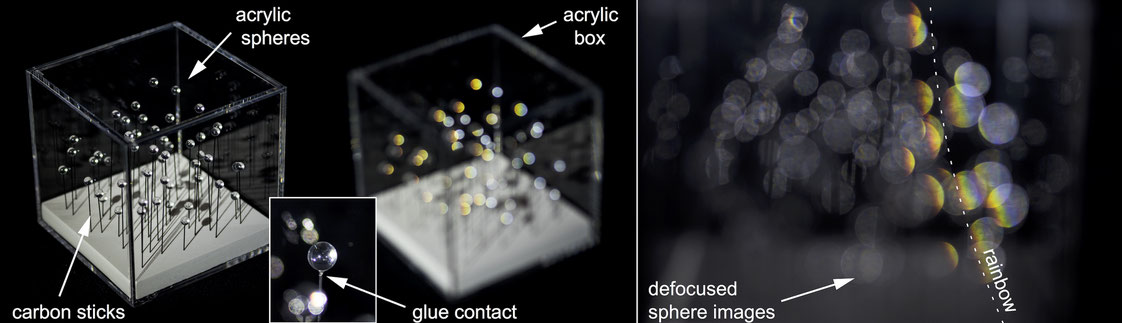
The aspect of the collective action of many scatterers partaking in the phenomenon of a perceived rainbows may be demonstrated by a stochastic arrangement of acrylic spheres in a 3D volume, mimicking the statistic distribution of raindrops in a rain shower. The approach is described in detail in the upcoming article "The rainbow in a box" accepted at the Am. J. Phys. (see publications). To this end, several acrylic spheres (diameter 6.4 mm) have been glued to black carbon sticks (diameter 0.5 mm) and mounted on a hard foam board and placed inside an acrylic box for protection. The spheres have been attached to the sticks using a helper device:
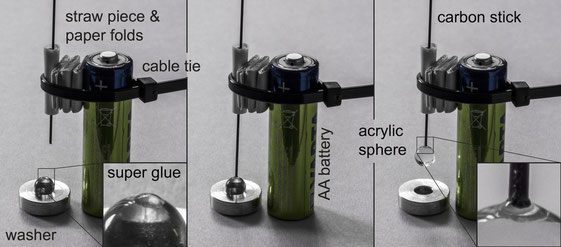
Preparation of the required sticks with mounted acrylic beads. These sticks were subsequently mounted into ~30 holes of a hard foam board of 11cm x 11cm x 11cm and placed into a box 12cm x 12 cm x 12 cm.
The finished demonstration setup in action is shown below. Upon illumination by a source of parallel white light (a bright 1000 lumen flashlamp) and photographed such that the camera, the box and the light source make an angle of about 15°, one can clearly see a rainbow segment. The focus was set far behind the box in the manual focus mode.
The dramatic effect of defocusing is comparable to the defocussing effect seen for a background of city night lights imaged in a portrait using a good "Bokeh"-lens:
In a future project we will extend this idea to mosaic halo projections using a volume of artificial glass crystals. Edmund Optics kindly sponsored this project with several hexagonal light pipes!
Alexander's dark band (or bright band?)
The vessel-wall effects (see text and figures further below) may also be observed using a water-filled cylindrical vase illuminated under an angle and thereby projecting the primary and the secondary rainbow on the supporting table. The alpha-like inner reflection component of the twinned primary rainbow appears as a faint offset rainbow inside Alexander's dark band. The rainbow angles and accordingly the width of Alexander's dark band between the primary and the secondary rainbow (beta-like contrast-rich outer reflection components) can be tuned by varying the incidence angle. The shallowest angle is closest to the natural rainbow phenomenon, while increasing incidence angles broaden the dark band. The tuning mechanism is the effective index of refraction determining the rainbow-like projected refraction in the 2D plane. This effective index of refraction is Bravais' index of refraction for inclined rays, which modifies the n_W=1.33 and n_G=1.47 to larger values with increasing inclination (for an accessible derivation see "Artificial Halos", Am. J. Phys., M. Selmke).
Alexander's dark band is not very easy to visualize with an artificial macroscopic raindrop (small liquid drops of 1-2mm can be used instead, cf. for instance the work of Jearl D. Walker). The reason lies in the near-field characteristics of the centuries old Florence's rainbow demonstration experiment. The embedded links hint at the reason. It turns out, that a large enough screen distance is required. Alternatively, acrylic spheres may be used (cf. for instance the cover image of the Am. J. Phys., Vol 83 No 9 or Harvard's experiment homepage note or even this youtube video: https://www.youtube.com/watch?v=HKsLh1yZVtE (beware of the conspiracy theories surrounding it, though)), although producing a much larger dark band (about 61°, cf. angle depicted in right-most sketch) and very different rainbow angles (1st order: 24°, 2nd order: 85°) as compared to the natural phenomenon (42° and 51°, and a 7.6° dark band between them).
The difference may be visualized via a ray tracing calculation, all in geometrical optics (i.e. Descartes' theory = spherical drop geometry + snell's law of refraction + ray bundleling close to the caustic + dispersion): The left images show the situation for an artificial raindrop, i.e. a 250mL round-bottom (Florence) flask filled with water (n=1.46 for the glass, n=1.33 for red light and water), with a wall-thickness of 1.7mm, for a 8.5cm flask diameter. Two zoom-levels are depicted. The rays have been transparency-coded according to their distance from the Cartesian rays (i.e. the rays experiencing the least deviation. 1st order: red, 2nd order: black. The inset spheres show the Cartesian rays).
Now, the right-hand image shows the situation for an acrylic sphere. Subscripts refer to the rainbow-order, superscripts to the ray-paths with outer reflections (beta) or inner reflections (alpha, cf. coated sphere rainbow scattering theory, commonly employed in liquid-cell refractometry). A's dark band for spheres without coatings is about 7.6°, while the effect of the wall is to reduce this width. For the depicted flask it is reduced to about 4°. The dark band emerges only beyond 1.22 meters.
The pictures below show the effect: The left-most image is a "magic (plant) growing jelly ball", which is a jelly-like sphere with a refractive index almost equal to that of water. One can clearly see the near-field bright band. (The distortions of the 2nd order bow in this case are likely due to the water meniscus at the bottom of the wet sphere / jelly ball. Putting the sphere on an absorbing piece of paper should remove the irregularity.)
The second image shows the Florence's rainbow demonstration experiment with the water-filled 250mL flask. The screens show projections on a screen (approx. 20x30cm) at positions (A), (B) and (C) in reference to the figure above. The third screen image shows the emergence of Alexander's dark band (screen distance: 1.5m). In the near-field, as with the jelly ball (water bead, left-most image), Alexander's dark band takes the form of a bright band.
For the acrylic sphere (last image), A's dark band is directly visible already close to the sphere (cf. raytracing above, or the cover image of the Am. J. Phys., Vol 83 No 9). All of this is beautifully explained by Descartes' almost 4 centuries-old theory...
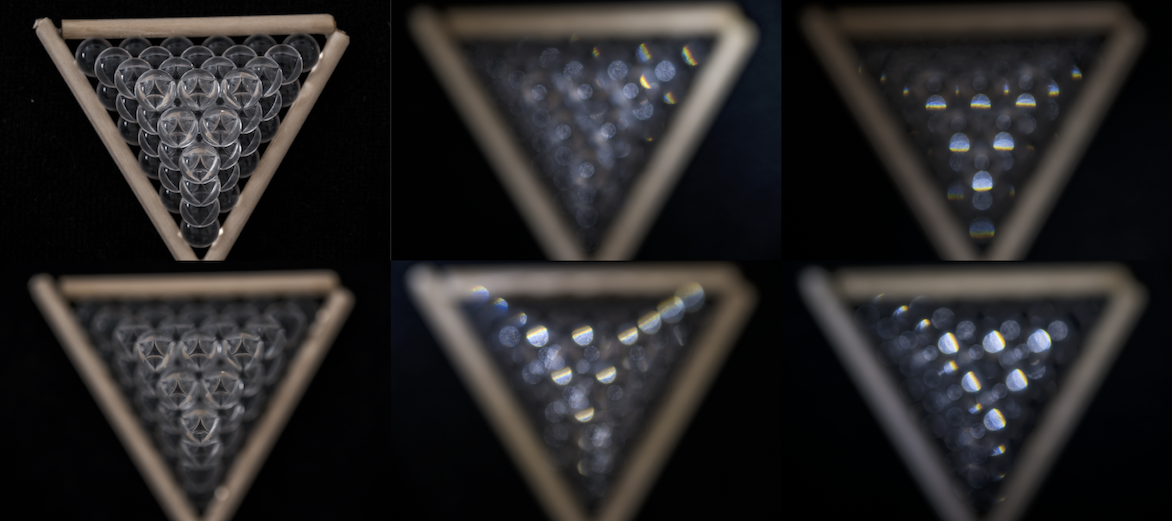
Photonic Crystals
With a batch of 100 acrylic spheres (6.4mm diameter, via www.modulor.de) I have created a small 3D photonic crystal. defocused imaging using a macro lens produces beautiful patterns. Close to the rainbow angle for acrylic glass (around 20°) spectrally dispersed reflexes appear. The crystal's structure causes regular patterns of reflexes to occur.
Artificial Parry arc (upper suncave Parry arc)
Following the same idea as below, one may find an artificial upper suncave Parry arc by the refraction through a cone, e.g. a water-filled Cosmopolitain cocktail / martini glass. Although the vertex angle is 70° instead of 60° as required for a perfect analogy, the shape is very similar and the raypath analogy still holds. To get a nice and clear Parry halo, one should either choose a very long projection distance l, or an obstructing mask (see image below).
Artificial Circumzenith and Circumhorizontal Arcs
Illuminating a cylindrical glass filled with water under a steep angle (for the circumhorizontal arc, CHA) or a shallow angle (for the circumzenith arc, CZA) produces analog ice halos. The CHA is distorted to a hyperbola in its projection on the vertical wall. Placing the glass in the sphere gives a truly horizontal arc. The average geometry of the (skew-)rays that produce these natural atmospheric optics phenomena are reproduced in this experiment. The average implied here is an average over plate crystal orientations, which together realize all skew-ray incidence angles occurring in the cylindrical refraction analogon.
The experiment itself appears long-known, however without a proper description/discussion of the phenomenon:
"Gilbert light experiments for boys", 1920, Experiment No. 94 "Artificial Rainbow", The A.C. Gilbert Company: https://archive.org/details/gilbertlightexpe00lynd
Also, in the 1798 book Practical Education Vol. 1, Maria Edgeworth and Richard Lovell Edgeworth 1798, London: one find the following passage on page 55-56: "S-, a little boy of nine years old, was standing without any book in his hand, and freely idle; he was amusing himself with looking at what he called a rainbow upon the floor: [...] The sun shone bright through the window; [...] At last he found, that when he moved the tumbler of water out of the place where it stood, his rainbow vanished. [...] immediately observed, that it was the water and the glas together that made the rainbow. [...]".
Many homepages can be found, e.g. by googling the terms "water glass rainbow", which (wrongly) describe the experiment as a demonstration of the rainbow phenomenon:
http://www.pbs.org/wgbh/nova/newton/lrk-act-rainbow.html
https://opticalillusion.wordpress.com/2008/07/21/how-to-make-a-rainbow-at-home/
http://lifestyle.iloveindia.com/lounge/how-to-make-a-rainbow-2613.html
http://www.asu.edu/courses/phs208/patternsbb/PiN/mod/light/opticsnature/pattLight4Obj1.html
http://www.nws.noaa.gov/os/educ/activit/rainbow.htm
http://redtri.com/how-to-make-a-rainbow/
http://littlebinsforlittlehands.com/how-to-make-rainbows-science-activities-kids/
http://www.scienceprojectideas.co.uk/making-rainbow-breaking-light-into-colour.html
http://www.kidsgen.com/school_projects/make_rainbow.htm
http://www.arvindguptatoys.com/arvindgupta/physicsexperiments.pdf
http://www.madsci.org/posts/archives/1999-01/916547183.Ch.r.html
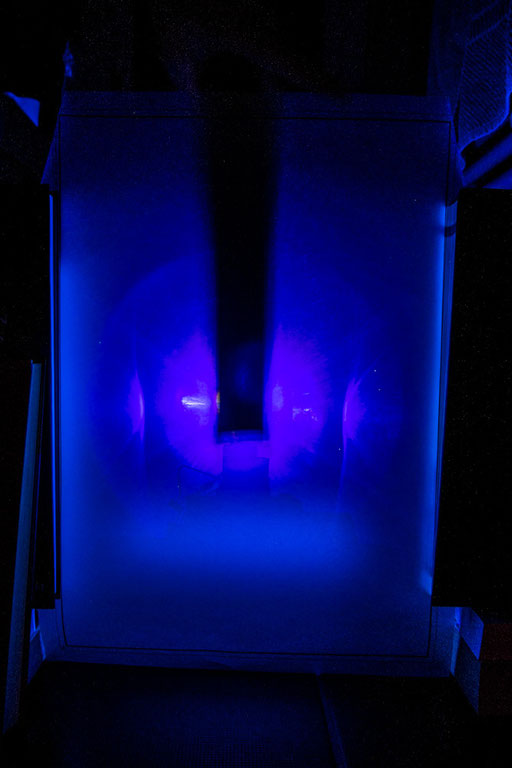
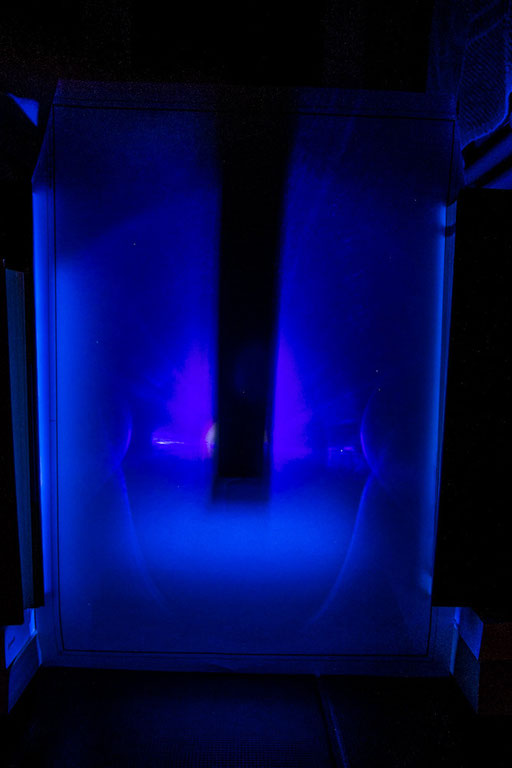
Spherical projection screen
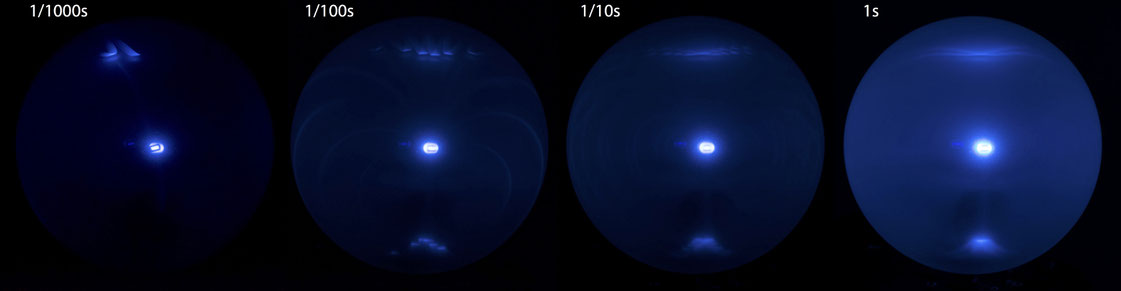
Finally done. (see also the BoredPanda.com article) Below you can see the results of the halo machine modules placed inside an acrylic 12" sphere (with a 3.5" opening at the bottom). The sphere was coated with a single layer of UV reactive (fluorescent) spray-paint. The laser diode was again a 403nm 20mW laser focusable diode. The violet you see in the pictures is the direct scattering from the UV laser diode, while the blueish color is fluorescence. The spherical screen technique generates artificial halos which are even closer to their natural counterparts which occur on the sky sphere.
the tangent arc is a fascinating fake caustic. The below sequence shows certain static configurations of the central motor, while the micro-motor is running. The accordingly reoriented "parhelic circles" are the components which blurr together to finally produce the tangent arcs:
An exposure sequence shows these components:
The parhelic circle, the Perry halo, the tangent arc halo and the Lowitz halo machine in operation:

The Lowitz machine in snapshots of 1/200s exposure showing the fake caustics constituents:
Map projections of halos
Since artificial halos from glass-crystals show larger minimum deviation angles, the halos angular extents are typically larger. This difference stems from the difference in the indices of refraction, 1.31 for ice as compared to about 1.51 for glass. An ideal projection screen that minimizes the distortion due to projection on a flat screen / wall is thus a spherical screen. Still, drawing the expected halos can give unsatisfactory results with standard projection techniques. The following images show a Mollweide Projection of the circumscribed Halo for ice and glass crystals.
Parry arcs

Parry arcs are produced by hexagonal columns oriented with the end faces vertical and one side-face lying flat. The following images were obtained rotating a glass rod in the same manner.
The zoomed in shapes show the peculiar form of the lower sunvex Parry arc for two different elevations of the incident light-source. The rain-bow colored bow is the upper suncave Parry arc and shows a clear color separation. For more information, see atoptics and meteros.de.
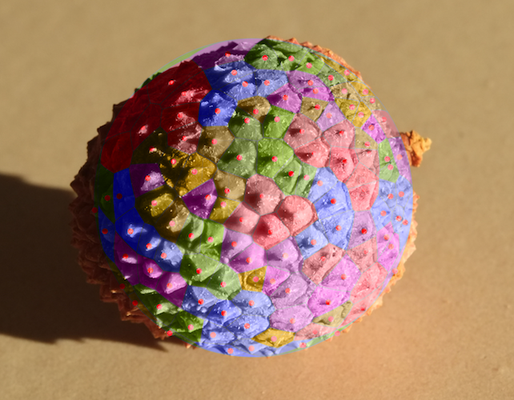
Lychee Voronoi tesselation
A special form of segmentation of space is the so-called Voronoi tesselation / Voronoi diagram. Given a a set of points, it subdivides the space into cells. Each cell is the subspace of the full 2D space in which the Euclidean distance is the shortest to the enclosed point from the point-set.
Lychee fruits seem to follow in their dragon-skin like pattern this segmentation. One may easily check this hypothesis by taking a picture of a Lychee fruit and using a free open-source Java-scipt tool. In biology, Infact, Voronoi tesselation and it's 3D counterpart are often recognized to describe cell patterns in tissue. However, seldomly is the reminiscence so striking and visible to the bare eye as in this case, I think.
A spherical geometry version of the Voronoi tesselation is more appropriate of course. An interactive applet may be found here.
Artificial complex halos

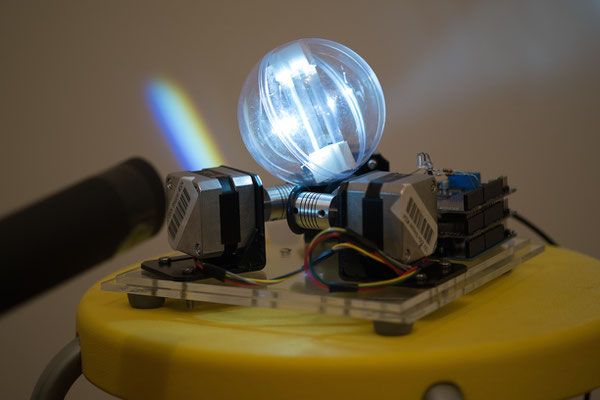
To create a complex halo display I have, together with my wife, constructed a versatile halo machine setup. A single motor drives 3 different subunits around a single axis.
- A simple plate-like crystal (polished side faces and top face) mounted on a screw and a shaft-coupler generates upon illumination the parhelic circle and in particular the embedded parhelia and the subparhelic cirlce with its subparhelia.
- A somewhat longer piece cut from the same 2mm wide hexagonal light homogenization rod serves as a rod-like crystal. It is mounted on a piece of acrylic (equipped with a shaft coupler) and glued to a tiny motor. When turned on and mounted on the larger motor it generates the tangential arc or the circumscribed halo. When the tiny motor is left off, the same construction allows the generation of Parry arcs.
- Finally, a small plate-like crystal piece glued on a corner to tiny motor on a similar construction allows the generation of Lowitz arcs. They cross right where the parhelia occur.
Combining all images (~20sec exposure, blue laser diode illumination) into a single image using Photoshop's blend mode "lighten", one arrives at the image shown on the right. Shown are two different light source elevations / inclinations. Note that the pictures contain some lens-flare from the central bright spot. These are the world's first complex artificial glas halos that have been produced... (referring to the comment/guestbook entry: "complex" refers to the combination of various halo types in a single display.)
These experiments expand upon the artificial recreation idea originally due to Auguste Bravais form 1847. Since then, similar experiments and machines have been constructed by A. Wegner, R. Greenler, M. Vollmer, R. Tammer, M. Großmann. The versatile and near-exhaustive modular approach adopted here simplifies the previous approaches and allows an easy implementation of everyone that is interested in the sciece of halos!

The parts used for these machines are mostly available from Conrad.de, e.g. the large and the small motor (Motoraxx XDrive K10WA, 2025-22), the shaft couplers, the acrylic piece (100x50x3mm) from which the bases were cut, the LiPo rechargable batteries (80mAh and 150mAh), wires, superglue and Gorilla plastic for the large motor base mount. The micro-switches from the previous version (cf. Lowitz machine vers. 1, see below) have been replaced by PH 2.0 JST 2-Pin female connector plugs and copper single core wire ended cable for a convenient plug connection and recharging ability with a LiPo charger (such as Adafruit's PowerBoost 500 Charger). The crystal pieces have been cut from a 2mm wide hexagonal glass rod obtained from Edmund Optics GmbH.
Using a spherical Lamp screen as a projection screen will emulate the sky and provide a more natural / equivalent result to the natural halo phenomenon. First results are promising.
The following sequence of images shows the setup in the tangent arc mode, i.e. using the second design of the 3 above and with all motors in operation. Starting with the most inclined illumination using a small Maglite flashlamp, one can see the evolution from an almost circumscribed halo to the tangent arc halo, see also Les Cowley's webpage for simulation images for ice crystals. Red light is refracted less and thus forms the inner edges. The apparent increasing distance of the tangent arc to the sun (light spot) is an artefact caused by the projection onto a plane wall instead on a spherical equidistant screen.
A complex artificial halo composition and its constituting images for white light illumination & dispersion:
Lowitz arc machine (vers. 1)

I have built a machine that rotates a small hexagonal BK7-glass crystal around two axes, specifically, the Lowitz axis and the c-axis. The device generates the upper and lower Lowitz arcs (see atmoptics.co.uk infopage) and also some x-reflection arcs going through the "sun". The illumination source is a blue 403nm laser diode.
Machine details:
I have used a small motor by (Motoraxx X-Drive 2025-22, 5700U/min, 1.5-3.0V, 0.5A) powered by a miniature 3.7V Lithium ion battery pack (80mAh). It is connected to the motor by a tiny switch and a multi-turn precision potentiometer with 50 Ohms. This was mounted (superglued) to a pice of metal onto which an axis coupler (2mm) was glued. This construction was mounted on another motor with a 2mm shaft which is powered by a somewhat larger Lithium ion battery pack, the same 50 Ohms potentiometer and a switch.
Lowitz arcs for glass (BK7): simulations
The Lowitz arcs for glass (n=1.51) are simuated using HaloSim on the right. Although the middle arc which extends almost vertically through the sundog position should exist, it is not observed in the experiment. The angular extent is rather large, such that physical obstructions are the likely cause for the non-observation.
In comparing the simulation to the experiments one should keep in mind that the artificial halo is projected onto a plane projection screen, whereas the simulation assumes a "projection" onto the sky sphere.

The current mechanical realization of the Lowitz machine is not yet fast enough to show an instant averaged intensity distribution. Instead, a quickly morphing projection of lines will be seen. The long-exposure (>20s) images show the final halo display, while the short exposure images (~2s) show multiple streaks, lines and loops (see also some of the images below).
The video on the left is approximately playing in real-time, demonstrating the effect. The rate of transitioning between those patterns varies over time, likely due to a mechanism akin to the Lissajous curves effect.
In contrast, a direct perception of the tangent and circumscribed arc could be seen, although a transitioning of brightness variations in the corresponding patterns of the tangent arc machine (see above) could also be observed.
Pyramidal ice crystal halo
The still pictures show a flashlight-illuminated water-filled bi-pyramidal acrylic crystal. Suspended on a wire, a long exposure image (8 sec) created the first artificial pyramidal ice crystal halo (see left). These halos a very rare but occur naturally, see http://www.atoptics.co.uk/halo/pyrpars.htm.
These halos have been predicted and computed by R.A.R. Tricker in his article "Arcs associated with halos of unusual radii", JOSA 69(8), p.1093-1100 (1979): Fig. 10-12.
Corresponding Monte Carlo simulations can also be found in W. Tape's book "Atmospheric Halos" in Fig. 10-18, p.93.
Artificial 22°-halo machine
The following images show a home-built Arduino-based Monte Carlo artificial halo machine. It uses three stepper motors to randomly reorient a hexagonal glass crystal placed inside a hollow acrylic sphere. White light transmitted through the setup produces a circular round halo when sufficient images are superimposed. The machine will be described in detail in an upcoming article (submitted). The article also contains an image of the generated artificial halo along with a movie showing the full sequence of random projections.
Caustics and refraction / reflections by various shapes
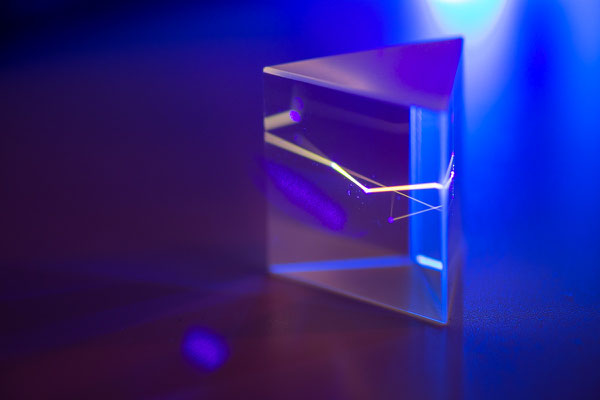
Caustics describe the diverging intensity of light due to the refraction of light impinging on a transparent object. Caustics occur near the envelope of a family of refracted rays. The following images show monochromatic and coloured caustics due to a sphere (including the 1st order, 2nd and 4th order rainbow caustics. The illuminated hexagon shows no caustics, but rotation will lead to a fake caustic (M. V. Berry, "Diffraction near fake caustics", Eur. J. Phys. 18, 303-306, 1997, home page of M. V. Berry: http://michaelberryphysics.wordpress.com). Refraction with dispersion causes the rainbow-like beams emerging from the prism, while color-dependent total internal reflection causes the blue beams to emerge from the prism when illuminated with approximately 30° inclination of the white light source.
Further images show refraction by a hexagonal prism made of BK7.
glass hexagon illuminated under 33° and 43° light source (flash lamp) elevation:
acrylic glass cubes illuminated by a focusable 405nm laser diode:
405nm laser diode illuminating various optics and glasses:
melting snow-flake clusters:
Poisson spot / spot of Arago
The "spot of Arago", also called the Poisson spot, is a phenomenon related to the wave-nature of light. Behind a perfectly opaque spherical object a shadow forms, in agreement with the naive expectation based on geometrical optics. However, the diffraction of light leads to the appearance of a bright spot in the center of the shadow, due to the constructive interference of waves emanating from the periphery of the illuminated sphere. This is the spot of Arago.
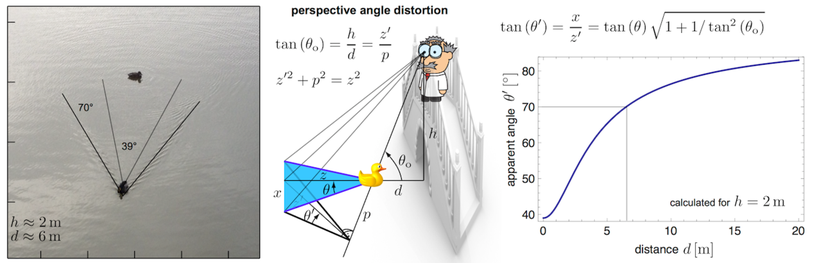
Duck wake
The full wake angle behind a Duck should be around 39°, according to the theory by Lord Kelvin. A recent study was published in 2013, "Ship Wakes: Kelvin or Mach Angle?", Physical Review Letters, by Marc Rabaud and Frédérik Moisy.
A short review of this article can be found on pro-physik.de.
I did a quick check on the laws of nature by taking a picture of a duck from a bridge in the Clara-Zetkin-Park in Sunday, 1st December 2013. Surprised at first by an apparent angle larger then 39°, the resolution is to take into account the perspective distortion of the angle when viewed from a distance, see image below! Indeed, the angle of roughly 70° in the picture is consistent with the situation under which the photo was shot.
Single water-drop rainbow

Inspired by the recent confirmed sighting of a tertiary rainbow (see sciencedaily.com) and by the wonderful paper by Jearl D. Walker "Multiple rainbows from single drops of water and other liquids" (Am. J. Phys. Vol. 44, No. 5, 1976) I have used a syringe to create a water drop and illuminated its periphery by a collimated laser beam from a laser-pointer to observe the subluminary rainbow interference pattern at the first rainbow angle of 42 degrees backwards..

Shown to the left is a photograph of the pattern which was reflected backwords by the water-drop. The radial intensity profile is plotted in the image, too. Theoretically described by "Debye Series for Gaussian beam scattering by a multilayered sphere", Renxian Li et al., 2007, Applied Optics, Vol. 46, No. 21, p. 4804. The plots in that paper are indeed comparable to the measured profile.
The experiment was done with a single wavelength illumination. A Rainbow is the same phenomenon with all the different wavelengths (colors) of the sun-light. Therefore, in order to observe a rainbow one should have the sun in ones' back and look towards an illuminated region of rain.
For a more detailed explanation check out: http://www.atoptics.co.uk/bows.htm
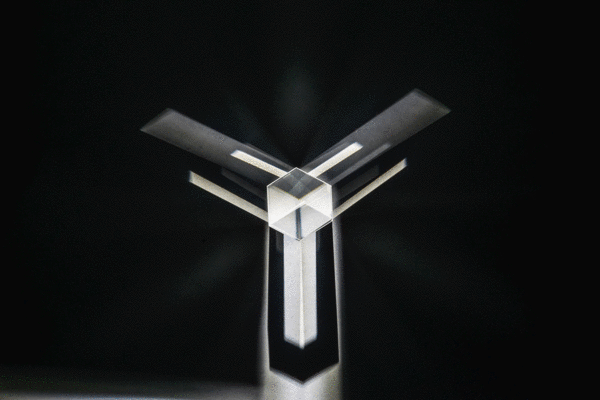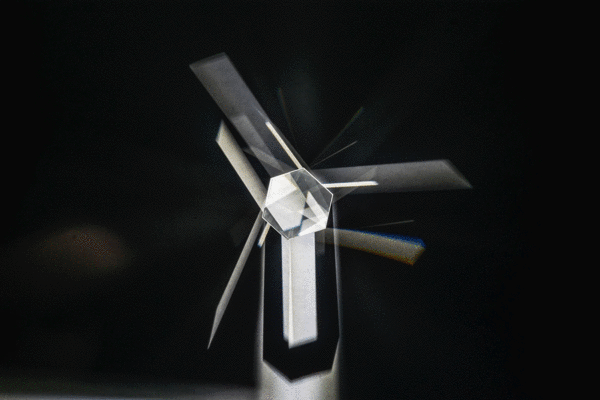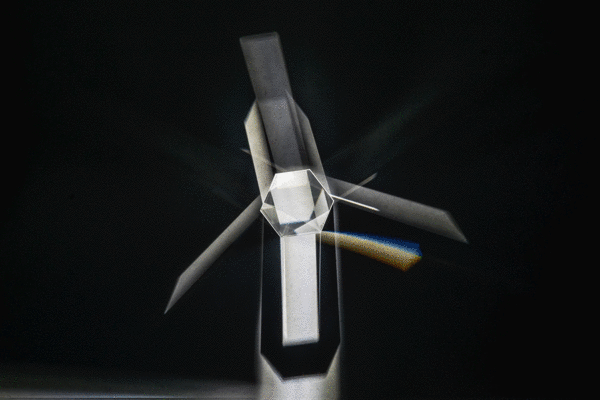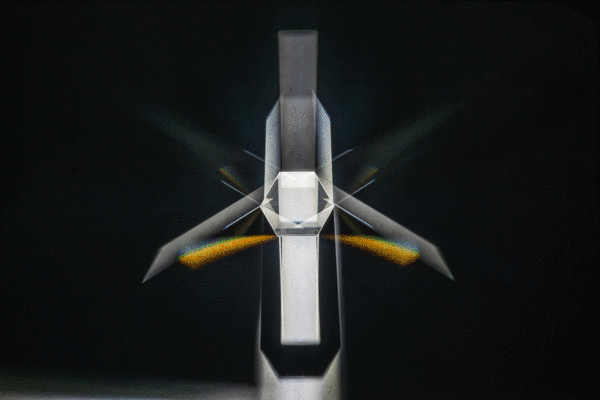
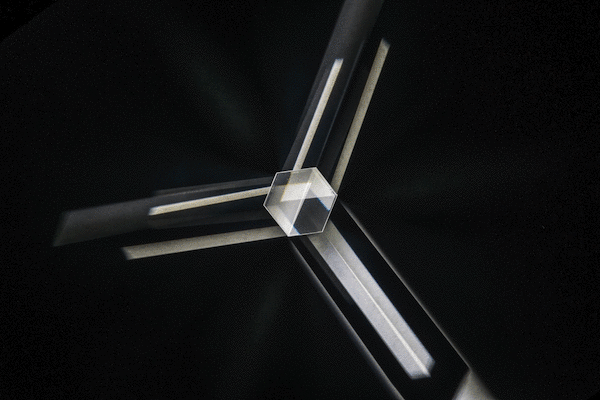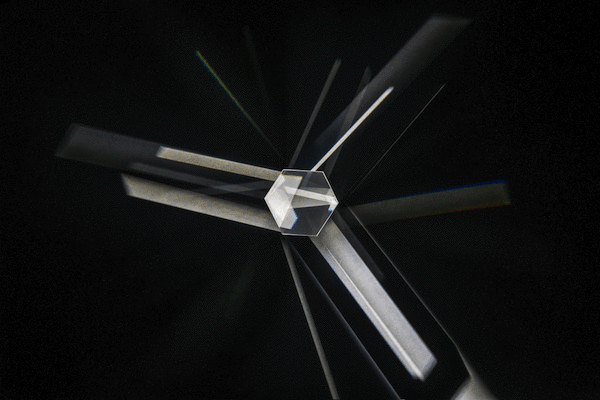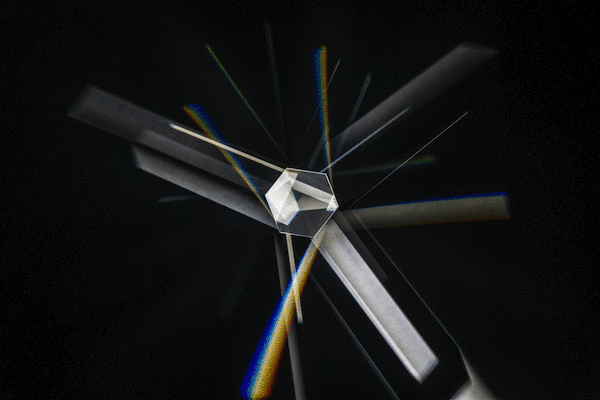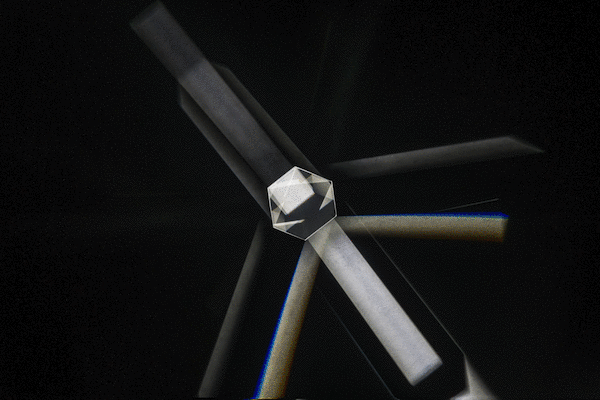
Michelson interferometer

The Michelson Interferometer played a major role in the discovery and formulation of Albert Einstein's theory of special special relativity (SRT). It proves the unchanging speed of light for coordinate-systems moving with a relative speed with respect to each other. A direct consequence, the Lorentz transformations, was a major step towards Einstein's groundbreaking theory.

The observable interference pattern after the recombination of the two split light-beams does not change upon reoriantation of the setup. This fact, in combination with the earth's revolution around the sun proves that the speed of the earth on its orbit does not add to the speed of light in our earth's moving reference frame.

A beam splitter cube was used to split the laser beam of a 488nm laser-pointer into two coherent beams. After reflection by a mirror, both beams are again superposed and the interference-pattern shows up on a screen.
Setup Components:

The setup uses a few mechanical components obtained from ebay and a surplus shop for optical elements (http://www.surplusshed.com/: beam splitters, high precision mirrors). The optomechanics were obtained from http://www.artifex-engineering.com/. The setups are protected from dust by custom dimensioned display cases from http://www.sora-shop.com/.
Gauss gun

This is a small multistage Gauss gun (nice illustration and building instruction here, or here) I have built. It shoots small metal spheres which aquire their kinetic energy from the conversion of potential energy of the magnetizable spheres in an inhomogeneous magnetic field (standard Neodym magnets) in a cascade-like fashion.